Mrs. Berg's Basics: January 2019

Monday, January 28, 2019

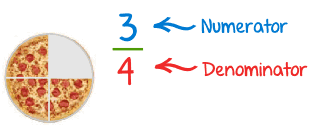
Comparing Fractions

We have moved into working on comparing fractions. There are numerous ways that we have learned to do this. Here are the ways that we have been working on:

Draw a picture. This strategy can work with smaller fractions, but starts to get complicated with larger fractions.

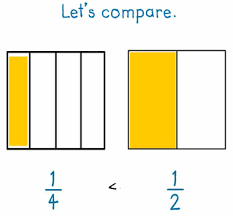
Compare with like denominators. When the denominators are the same, you are comparing the numerator. The larger numerator will be the larger fraction.

$Image result for compare fractions with like denominators$

Compare with like numerators. When the numerators are the same, you are comparing the denominator. The larger the denominator, the smaller the pieces will be. Therefore, the smaller denominator will give you the larger fraction.

Compare to a benchmark fraction. Determine how the fraction relates to ½ and that can help determine the larger or smaller fractions. We have also put them on a number line to help us clearly see how they compare.

Compare missing pieces. The fraction with the smallest piece missing will be the largest fraction.

$visual image of fractions$

Change one denominator to make a common denominator. Sometimes you only have to change one of the denominators to make common denominators.

Change both denominators to a common denominator. This allows kiddos to then compare fractions with like denominators. These are a few strategies we have talked about relating to this:

Change both denominators to a common denominator.

Multiply the denominators by each other to find a common denominator.

Find the least common denominator. Finding the LCD has kiddos finding the least common multiple/product (the smallest positive number that is a multiple of two or more numbers).

Steps to find the LCD (Least Common Denominator)

Example: Compare 1/2, 1/3, 1/5

1. Identify the denominators.

2, 3, 5

2. Find the Least Common Multiples (LCM) of the three denominators. The LCM is also the Least Common Denominator (LCD).

Multiples of 2: 2; 4; 6; 8; 10
Multiples of 3: 3; 6; 9; 12; 15
Multiples of 5: 5; 10; 15; 20; 25

Note that if no common denominator exists at this point, you may need to continue writing out multiples until you eventually come across a shared multiple.
Example: 2 x 15 = 30; 3 x 10 = 30; 5 x 6 = 30
The LCD = 30

3. To write equivalent fractions, multiply the numerator by the same number the denominator was multiplied by to get the common denominator.

Example: 15 x (1/2); 10 x (1/3); 6 x (1/5)

New mathematical statement: 15/30 > 10/30 > 6/30

Here are some games to help solidify these skills:

Battleship Numberline

Thinking Blocks

Fractions App

Please let me know if you have any questions.

Monday, January 14, 2019

Equivalent Fractions

We are full swing into our unit on fractions. We are working on solidifying how to compose equivalent fractions. We have a few strategies for finding equivalent fractions (There are links to lessons underneath each strategy):

1. use fraction bars (These are hands on manipulatives that we use in class. I also have a paper copy if you would like a set to cut out and use at home.)

$Image result for equivalent fractions with fraction bars$

Interactive Fraction Bars

2. draw fraction strips (This is something that they can do to already existing pictures or they can draw themselves.)

$Image result for equivalent fractions with fraction bars$

Equivalent Fraction Strips Lesson

3. use multiplication chart

Equivalent Fractions Using a Multiplication Chart

3. multiply or divide numerator and denominator by 1 (3/3, 5/5, 10/10)

Here are some games that will help further these skills:

Equivalent Fractions PacMan

Equivalent Fractions Concentration

Remind your kiddo that in order for the fraction to be equivalent, what you do to the numerator MUST be done to the denominator as well. Please let me know if you have any questions!

Have a great week!

Tuesday, January 8, 2019

Properties of Soil

Soil is a mixture of rock, organic material, water, and air in which plants can grow. We study three main types of soil; sand, loam, and clay. We also work with humus.

Sand has large, loose grains, few nutrients, and does not hold water well which washes out nutrients so that it does not support plant growth. This type of soil does not have well developed horizons.

Loam is a mix of sand, silt, and clay. Most potting soil is loamy as it is rich in humus and holds water better than sand so it remains wet longer during dry periods. Loamy soils have a thick topsoil horizon.

Clay has closely pack particles which means there is very little air space. The particles are extremely fine and powdery. It is rich in nutrients and holds lots of water, but doesn't soak it in quickly.

Humus is the remains of decayed plants and animals, which contains nutrients that plants need to grow. It also helps the soil to retain moisture.

This website allows me to learn about the different types of soil and learn more than just the names. I think the student's need to know the differences but first I need to ensure I know them and can answer questions about them, and I think this website will help.

Image result for types of soil 4th grade

When soil forms, it develops layers, called soil horizons. Scientists use a letter to identify each soil horizon. A vertical section of soil that shows the layers is called a soil profile.

Here are some games that can take this concept further:

Soil Games

Watch some funny videos here:

Types of Soil

Soil Profile

Here are some places to go for more information:

Properties of Soil

Fraction & Decimal Connections

Fraction and Decimal Connections

$Image result for fractions and decimals$

We are working on the connections between fractions and decimals. Decimals and fractions represent the same thing: a number that is not exactly a whole number. To convert fractions to decimals, decimals need to have a 10 or 100 in the denominator. Then you just plug it into the correct place value.

$Image result for fractions to decimals$

So,

$Image result for fractions decimals and money$

Here are some games that will help practice this skill:

Model Decimals and Fractions

Matching Game

Puppy Chase

Please let me know if you have any questions.